POMDP Formulation Approximations

Recap

  • Alpha Vectors
  • Best solver for discrete POMDPs:

POMDP Computational Complexity

  • Infinite horizon POMDPs are undecidable
  • Finite horizon POMDPs are PSPACE Complete
    • Among the hardest problems that can be solved using a polynomial amount of space
    • Any algorithm that can solve a general POMDP will have exponential complexity

(we think)

Sad facts 😭

Approximate POMDP Solutions

Numerical Approximations

(approximately solve original problem)

Offline

Online

Formulation Approximations

(solve a slightly different problem)

Last week

Thursday

Today!

Rotor Failure Example

POMDP Objective

\[\pi^*  = \underset{\pi : B \to A}{\text{argmax}} \,\, \text{E}\left[\sum_{t=0}^\infty \gamma^t R(s_t, \pi(b_t))\right]\]

\[b' = \tau(b, a, o)\]

Certainty Equivalent

\[\pi^*  = \underset{\pi : B \to A}{\text{argmax}} \,\, \text{E}\left[\sum_{t=0}^\infty \gamma^t R(s_t, \pi(b_t))\right]\]

\[b' = \tau(b, a, o)\]

POMDP Objective

\[\pi_{\text{CE}}(b)  = \pi_s (\underset{s\sim b}{\text{E}[s])}\]

\[b' = \tau(b, a, o)\]

Certainty Equivalent

Optimal for LQG

QMDP

\[\pi^*  = \underset{\pi : B \to A}{\text{argmax}} \,\, \text{E}\left[\sum_{t=0}^\infty \gamma^t R(s_t, \pi(b_t))\right]\]

\[b' = \tau(b, a, o)\]

POMDP Objective

\[\pi_\text{QMDP}(b)  = \underset{a \in A}{\text{argmax}} \,\, \underset{s\sim b}{\text{E}}\left[Q_\text{MDP}(s, a)\right]\]

\[b' = \tau(b, a, o)\]

Example: Tiger POMDP with Waiting

\begin{aligned} & \mathcal{S} = \mathbb{Z} \quad \quad \quad ~~ \mathcal{O} = \mathbb{R} \\ & s' = s+a \quad \quad o \sim \mathcal{N}(s, s-10) \\ & \mathcal{A} = \{-10, -1, 0, 1, 10\} \\ & R(s, a) = \begin{cases} 100 & \text{ if } a = 0, s = 0 \\ -100 & \text{ if } a = 0, s \neq 0 \\ -1 & \text{ otherwise} \end{cases} & \\ \end{aligned}

State

Timestep

Accurate Observations

Goal: \(a=0\) at \(s=0\)

Optimal Policy

Localize

\(a=0\)

POMDP Example: Light-Dark

POMDP Solution

QMDP

Same as full observability on the next step

Information Gathering

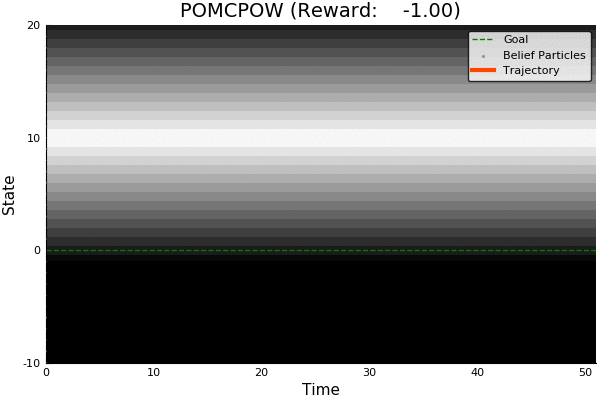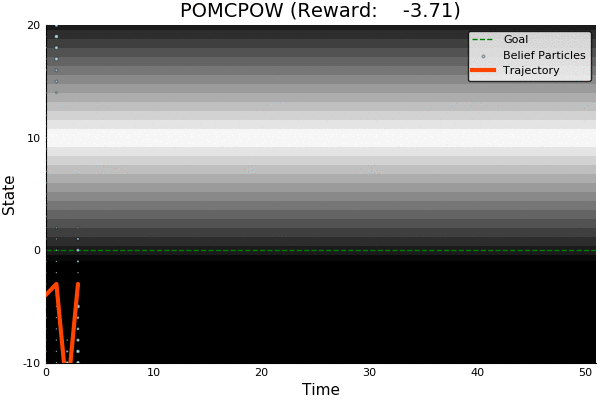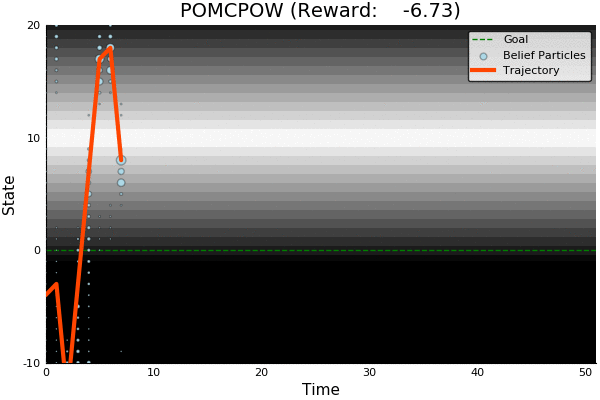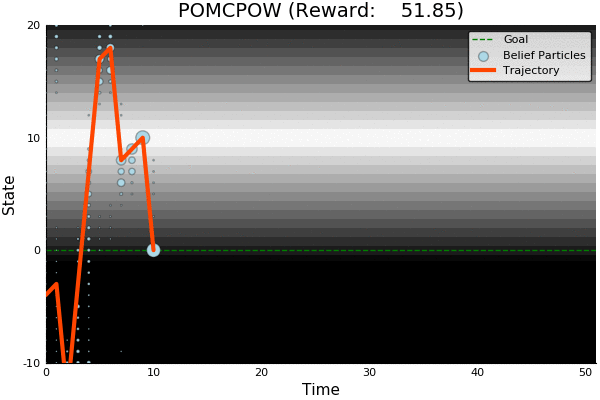
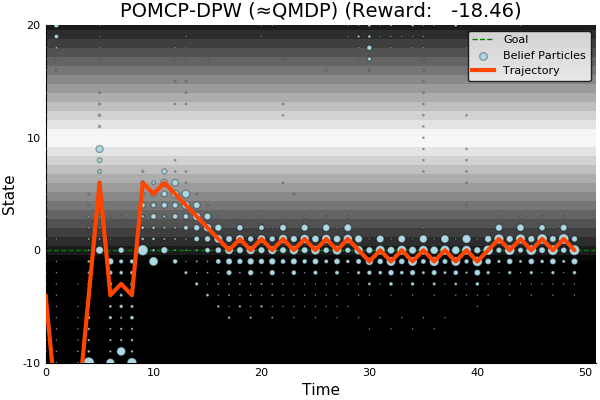
QMDP

Full POMDP

QMDP

INDUSTRIAL GRADE

QMDP

ACAS X

[Kochenderfer, 2011]

Hindsight Optimization

\[\pi^*  = \underset{\pi : B \to A}{\text{argmax}} \,\, \text{E}\left[\sum_{t=0}^\infty \gamma^t R(s_t, \pi(b_t))\right]\]

\[b' = \tau(b, a, o)\]

POMDP Objective

FIB

\[\pi^*  = \underset{\pi : B \to A}{\text{argmax}} \,\, \text{E}\left[\sum_{t=0}^\infty \gamma^t R(s_t, \pi(b_t))\right]\]

\[b' = \tau(b, a, o)\]

POMDP Objective

k-Markov

\[\pi^*  = \underset{\pi : B \to A}{\text{argmax}} \,\, \text{E}\left[\sum_{t=0}^\infty \gamma^t R(s_t, \pi(b_t))\right]\]

\[b' = \tau(b, a, o)\]

POMDP Objective

Open Loop

\[\pi^*  = \underset{\pi : B \to A}{\text{argmax}} \,\, \text{E}\left[\sum_{t=0}^\infty \gamma^t R(s_t, \pi(b_t))\right]\]

\[b' = \tau(b, a, o)\]

POMDP Objective